
Statement (1) should get you thinking about the 5-12-13 right triangle, as it tells us that the hypotenuse is 13. Now that we know what information we need for sufficiency, let’s examine each statement on its own.
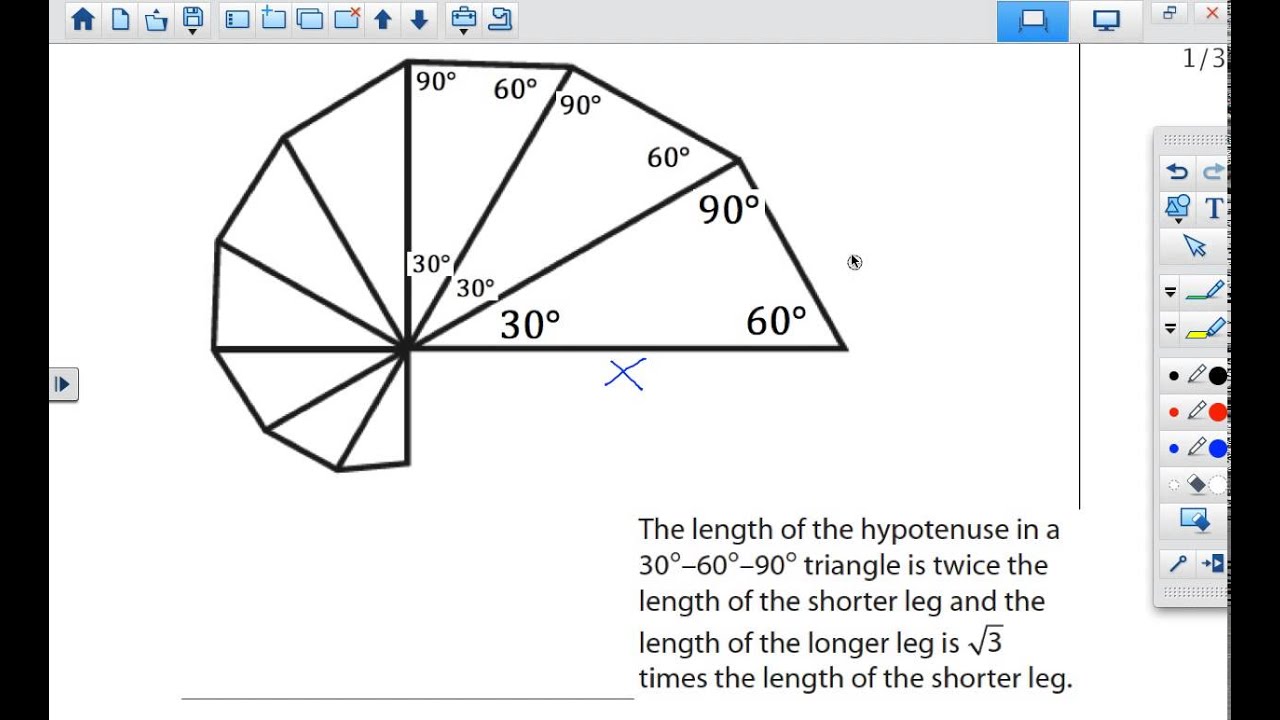
You can save yourself much time and mental energy by having a solid idea of what information you need from the statements for sufficiency before you actually view the statements. For example, on a different problem with the same question, if the test had said that the product of the base and height were 30, that would have been sufficient, as that would be enough for us to deduce that the area is 15. Or, as a matter of fact, we could have sufficiency if we knew xy as a product, even if we didn’t know the values of x and y, individually.
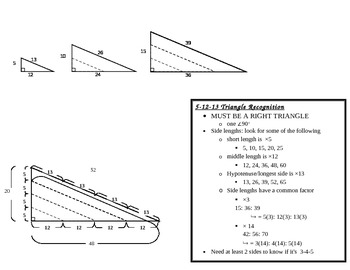
We need to know the individual values of x and y. Remember, it helps to frame Data Sufficiency questions in terms of what information you need to get to the answer. That means A = xy /2, since x and y represent the height and base, respectively. A represents the area of the triangle, which is found by multiplying base by height and dividing by 2. Give it a try on your own before reading any further.Īs with any Data Sufficiency question, let’s identify what we’re asked to find. If A is the area of a triangle with sides of lengths x, y, and z as shown above, what is the value of A ? Let’s take a look at an Official Guide Data Sufficiency problem that illustrates how the test can force you to engage some higher-level reasoning skills: These work because if you check the arithmetic, 5 ² + 12 ² = 13 ² and 7 ² + 24 ² = 25 ² :Īs we’ve continually discussed, however, your success on more difficult GMAT problems will require you to go beyond mere rote memorization. So now we come to the main topic: what are some other common “Pythagorean triples” the GMAT may test? The next base triples that fit the Pythagorean Theorem are 5-12-13 and 7-24-25. No matter what positive integer n you choose for the figure above, you will produce a valid right triangle. This works not only for 3-4-5 but also for 6-8-10, 9-12-15, or any other multiples of each side length. You’ll recall that the Pythagorean Theorem ( a² + b² = c² ) holds for any right triangle where a and b are the two legs and c is the hypotenuse, and that the 3-4-5 triangle represents the smallest such triangle with all integer side lengths: Date: 12th January, 2021 The 5-12-13 and 7-24-25 Right TrianglesĪlthough the 3-4-5 right triangle is by far the most common of the so-called “Pythagorean triples” tested on the GMAT, there are a few others worth knowing.


 0 kommentar(er)
0 kommentar(er)
